Dr. J's Maths.com
Where the techniques of Maths
are explained in simple terms.

Trigonometry - Solving equations.
Test Yourself 2.


 

The questions below focus on the formats:
1. Trig ratio and a constant.
2. Squared trig ratio and a constant.
3. Sin and cos terms but no constant.
4. Trig ratios in a quadratic format.
5. Using angles of any magnitude.
6. Number of solutions.

 

1. Trig ratio and a constant.

(solve all equations for
0 ≤ angle ≤ 360°).

 1. 2. .
Answer.120°, 240°.
3. .
Answer.30°, 60°, 210°, 240°. 		4.
  5. 6. Solve 2sin θ cot θ + 1 = 0
Answer.0°, 180°, 360°, 120°, 240°.
  7. sin β = -0.6691.
Answer.β = 222°, 318°.		 8.
  9. . 10.
2. Squared trig ratio and a constant. 11.  
  13.  
  15.  
3. Sin and cos terms but no constant. 17. sinx = cos(2x - 45°).
Answer.45°.		 18. cos 3x = sin (x - 22°).
Answer.56°.
  19. sec x - 4cos x = 0
Answer.60°, 120°, 240°, 300°.		 20. Find θ if sin(θ + 20°) = cos (2θ - 50°).
  21. 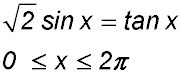. 22.
 

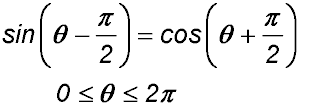23. (i) Simplify tan2A(1 - sin2A).

(ii) Hence or otherwise solve

tan2A(1 - sin2A) for 0 ≤ θ ≤ 360°)

Answer.90°, 270°.		 24.
4. Trig ratios in a quadratic format. 25. Solve 2cos2 x - 3 cos x + 1 = 0
Answer.0°, 60°, 300°, 360°.		 26. Solve 3tan4 x + 2tan2 x - 1 = 0
Answer.30°, 150°, 210°, 330°.
  27. 3 sec2 x - tanx - 5 = 0
Answer.45°, 146°19', 225°, 326°19'.		 28. 2cot2x - cosecx + 1 = 0
Answer.90°.
  29. 3 sinx - 2 tanx = 0
Answer.0°, 48° 11', 180°, 311° 49'.		 30. (i) Simplify 2cos2 X + 3sin2 X - 2.

(ii) Hence or otherwise solve:

2cos2 α + 3sin2 α - 2 = 0

Answer.(1) sin2α.
(ii) 90°, 270°.

5. Complementary or supplementary angles.

(0 ≤ θ ≤ 360°).

 31. tan (3x + 15°) = cot (5x - 29°).
Answer.13°.		 32. Given 3cos θ + 2 = 0 and that
tan θ > 0, find the value of tan (θ - 180o).
     
6. Number of solutions.

Find the solutions for

(2sinx + 1)(cos x - 3) = 0.

Answer.210° and 330°.