Where the techniques of Maths
are explained in simple terms.
Financial Maths - Annuities and Present value tables.
Background briefing.
- Algebra & Number
- Calculus
- Financial Maths
- Functions & Quadratics
- Geometry
- Measurement
- Networks & Graphs
- Probability & Statistics
- Trigonometry
- Maths & beyond
- Index
The future value of an annuity can be regarded as being
the amount of money which will accumulate
as the result of the investment of regular annuity payments throughout a specified future time.
The present value of an annuity can be regarded as being
the amount of money which might be invested now to allow
the payment of an annuity of a certain amount throughout a given future time.
Hence in the Present Value situation, it is the result of an annuity paid over a number of periods and then brought back to the present value of that annuity.
The concept of a present value therefore is in some ways the reverse of the future value concept.
| Future value | Present value | |
| emphasises how much regular payments over a set number of periods will amount to at a given time in the future. | emphasises the single amount we need to deposit now into a compound interest account to enable a regular annuity to be paid. |
The underlying rationale for the difference is that, as time passes, an amount of money received now is worth more than the same sum at a future date. So $10 received now will buy more than $10 in the future. The value (or buying power) of a given amount of money decreases over time.
There are two types of situations involving Present Value calculations:
- the calculation of the amount to be deposited now so as to be able to make regular payments for a reasonable time into the future.
This type of calculation is really only the usual compound interest calculation and it has been used previously.
- the determination of the amounts for each regular payment in an annuity given the amount of funds in an investment account.
For questions of the first type just involving compound interest, the relationship is expressed as
Future value = Present value × Compound interest rate
FV = PV × (1 + r)n
where r = interest rate paid on the deposit
n = number of periods for the investment.
This relationship can be rewritten to make the PRESENT VALUE the subject of the equation:
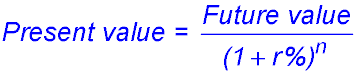
The more commonly addressed question relates to an annuity being paid from an investment amount on a regular basis (as for old people drawing down their superannuation accounts each month or injured workers and sports people who need to draw on a savings or investment account).
People in such circumstances withdraw funds and so their subsequent interest is paid on a decreasing balance in the fund. Hence we often hear of people asking questions as to how long they can sustain regular payments given assumptions about the interest rates and the amount a person wishes to withdraw on a regular basis.
In these situations, there are constant withdrawals (and so it is an annuity). The above relationship is too simplistic because it does not address the amount of withdrawals. Tables have therefore been prepared which give interest factors which can be applied to the withdrawal amounts - just like the interest factors for Future values can be applied to investment annuities.

Present Value tables are included elsewhere.
There are many questions which can be asked about present values. Many of these focus on investments and annuities but others focus on loans such as how much can be borrowed to buy something such as a computer or a car.
A typical question asked about regular payments deducted from an investment account is as follows:
How much money should be in an annuity fund to enable $500 to be withdrawn each month for 6 years? The fund pays interest at 6% p.a.
For such questions a Table of Present values will always be provided. Part of such a table is as follows:
| Periods | 0.5% | 0.6% | 1% |
| 6 | 5.8964 | 5.8760 | 5.7955 |
| 60 | 51.7256 | 50.2621 | 44.9550 |
| 72 | 60.3395 | 58.3253 | 51.1504 |
To answer this question, follow these steps:
| 1. convert the annual interest rate to the one relevant to the question. |
6% p.a. converts to 0.5% p.m |
| 2. Determine the number of periods. | In 6 years there are 72 months. |
| 3. Look up the table for that interest % and the number of periods. |
Here for 72 periods and 0.5%, the table value is 60.3395 |
| 4. Substitute into the relationship shown above in blue with the annuity amount. |
Present value = 500 × 60.3395 = $30,170 |
Hence the fund needs $500 × 60.33951 = $30,170 in it now to enable monthly $500 withdrawals for 6 years.
The question can be asked in reverse also. For example, given a present amount in an annuity account, what withdrawal could be made to span a given period of time? Examples of both types are included in the Test Yourself questions.