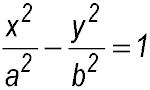Dr. J's Maths.com
Where the techniques of Maths
are explained in simple terms.
Where the techniques of Maths
are explained in simple terms.
Calculus - Differentiation - Implicit Differentiation.
Test Yourself 1.
- Algebra & Number
- Calculus
- Financial Maths
- Functions & Quadratics
- Geometry
- Measurement
- Networks & Graphs
- Probability & Statistics
- Trigonometry
- Maths & beyond
- Index
| Direct differentiation. | Use implicit differentiation to find
|
| Derive the equation of the following hyperbola
|
|
| Find the derivative of y2 = x2y + 1.
|
|
| Stationary points. | Find the coordinates of any stationary points for the curve
y3 + 2xy + x2 + 2 = 0.
|
| Find the coordinates of the points where the tangent to the curve x2 + 2xy + 3y2 = 8 is horizontal.
|
|
| Gradients. | Find the equation of the tangent to the curve defined by
x2 - xy + y3 = 5 at the point (2, -1).
|
| Find the coordinates of the point where the tangent to the curve x2 - y2 + xy + 5 = 0 is parallel to the line y = x.
|
|
| A plane curve is defined implicitly by the equation
x2 + 2xy + y5 = 4. This curve has a horizontal tangent at P (x, y). Show that x is a root of the equation x5 + x2 + 4 = 0. |